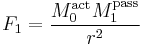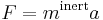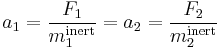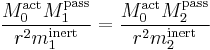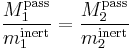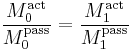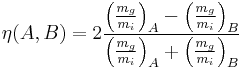Equivalence principle
| General relativity |
|---|
| Introduction Mathematical formulation Resources |
|
Fundamental concepts
|
|
Phenomena
|
|
Advanced theories
|
In the physics of general relativity, the equivalence principle is any of several related concepts dealing with the equivalence of gravitational and inertial mass, and to Albert Einstein's assertion that the gravitational "force" as experienced locally while standing on a massive body (such as the Earth) is actually the same as the pseudo-force experienced by an observer in a non-inertial (accelerated) frame of reference.
Einstein's statement of the equivalence principle
A little reflection will show that the law of the equality of the inertial and gravitational mass is equivalent to the assertion that the acceleration imparted to a body by a gravitational field is independent of the nature of the body. For Newton's equation of motion in a gravitational field, written out in full, it is:
It is only when there is numerical equality between the inertial and gravitational mass that the acceleration is independent of the nature of the body.
- (Inertial mass)
(Acceleration)
(Intensity of the gravitational field)
(Gravitational mass).
— Albert Einstein, [1]
Development of gravitation theory
Something like the equivalence principle emerged in the late 16th and early 17th centuries, when Galileo expressed experimentally that the acceleration of a test mass due to gravitation is independent of the amount of mass being accelerated. These findings led to gravitational theory, in which the inertial and gravitational masses are identical.
The equivalence principle proper was introduced by Albert Einstein in 1907, when he observed that the acceleration of bodies towards the center of the Earth at a rate of 1g (g = 9.81 m/s2 being a standard reference of gravitational acceleration at the Earth's surface) is equivalent to the acceleration of an inertially moving body that would be observed on a rocket in free space being accelerated at a rate of 1g. Einstein stated it thus:
we [...] assume the complete physical equivalence of a gravitational field and a corresponding acceleration of the reference system.—Einstein, 1907
That is, being at rest on the surface of the Earth is equivalent to being inside a spaceship (far from any sources of gravity) that is being accelerated by its engines. From this principle, Einstein deduced that free-fall is actually inertial motion. Objects in free-fall really do not accelerate, but rather the closer they get to an object such as the Earth, the more the time scale becomes stretched due to spacetime distortion around the planetary object (this is gravity). An object in free-fall is in actuality inertial, but as it approaches the planetary object the time scale stretches at an accelerated rate, giving the appearance that it is accelerating towards the planetary object when, in fact, the falling body really isn't accelerating at all. This is why an accelerometer in free-fall doesn't register any acceleration; there isn't any. By contrast, in Newtonian mechanics, gravity is assumed to be a force. This force draws objects having mass towards the center of any massive body. At the Earth's surface, the force of gravity is counteracted by the mechanical (physical) resistance of the Earth's surface. So in Newtonian physics, a person at rest on the surface of a (non-rotating) massive object is in an inertial frame of reference. These considerations suggest the following corollary to the equivalence principle, which Einstein formulated precisely in 1911:
Whenever an observer detects the local presence of a force that acts on all objects in direct proportion to the inertial mass of each object, that observer is in an accelerated frame of reference.
Einstein also referred to two reference frames, K and K'. K is a uniform gravitational field, whereas K' has no gravitational field but is uniformly accelerated such that objects in the two frames experience identical forces:
We arrive at a very satisfactory interpretation of this law of experience, if we assume that the systems K and K' are physically exactly equivalent, that is, if we assume that we may just as well regard the system K as being in a space free from gravitational fields, if we then regard K as uniformly accelerated. This assumption of exact physical equivalence makes it impossible for us to speak of the absolute acceleration of the system of reference, just as the usual theory of relativity forbids us to talk of the absolute velocity of a system; and it makes the equal falling of all bodies in a gravitational field seem a matter of course.—Einstein, 1911
This observation was the start of a process that culminated in general relativity. Einstein suggested that it should be elevated to the status of a general principle when constructing his theory of relativity:
As long as we restrict ourselves to purely mechanical processes in the realm where Newton's mechanics holds sway, we are certain of the equivalence of the systems K and K'. But this view of ours will not have any deeper significance unless the systems K and K' are equivalent with respect to all physical processes, that is, unless the laws of nature with respect to K are in entire agreement with those with respect to K'. By assuming this to be so, we arrive at a principle which, if it is really true, has great heuristic importance. For by theoretical consideration of processes which take place relatively to a system of reference with uniform acceleration, we obtain information as to the career of processes in a homogeneous gravitational field.—Einstein, 1911
Einstein combined the equivalence principle with special relativity to predict that clocks run at different rates in a gravitational potential, and light rays bend in a gravitational field, even before he developed the concept of curved spacetime.
So the original equivalence principle, as described by Einstein, concluded that free-fall and inertial motion were physically equivalent. This form of the equivalence principle can be stated as follows. An observer in a windowless room cannot distinguish between being on the surface of the Earth, and being in a spaceship in deep space accelerating at 1g. This is not strictly true, because massive bodies give rise to tidal effects (caused by variations in the strength and direction of the gravitational field) which are absent from an accelerating spaceship in deep space.
Although the equivalence principle guided the development of general relativity, it is not a founding principle of relativity but rather a simple consequence of the geometrical nature of the theory. In general relativity, objects in free-fall follow geodesics of spacetime, and what we perceive as the force of gravity is instead a result of our being unable to follow those geodesics of spacetime, because the mechanical resistance of matter prevents us from doing so.
Since Einstein developed general relativity, there was a need to develop a framework to test the theory against other possible theories of gravity compatible with special relativity. This was developed by Robert Dicke as part of his program to test general relativity. Two new principles were suggested, the so-called Einstein equivalence principle and the strong equivalence principle, each of which assumes the weak equivalence principle as a starting point. They only differ in whether or not they apply to gravitational experiments.
Modern usage
Three forms of the equivalence principle are in current use: weak (Galilean), Einsteinian, and strong.
The weak equivalence principle
The weak equivalence principle, also known as the universality of free fall or the Galilean equivalence principle can be stated in many ways.
- The trajectory of a point mass in a gravitational field depends only on its initial position and velocity, and is independent of its composition.
- All test particles at the alike spacetime point in a given gravitational field will undergo the same acceleration, independent of their properties, including their rest mass.[2]
- All local centers of mass vacuum free fall along identical (parallel-displaced, same speed) minimum action trajectories independent of all observable properties.
- The vacuum world line of a body immersed in a gravitational field is independent of all observable properties.
- The local effects of motion in a curved space (gravitation) are indistinguishable from those of an accelerated observer in flat space, without exception.
- Mass (measured with a balance) and weight (measured with a scale) are locally in identical ratio for all bodies (the opening page to Newton's "Principia").
Locality eliminates measurable tidal forces originating from a radial divergent gravitational field (e.g., the Earth) upon finite sized physical bodies. The "falling" equivalence principle embraces Galileo's, Newton's, and Einstein's conceptualization. Relativistic particles and photons do not fall like local masses. Photons in a gravitational field experience twice the local gravitational acceleration (tracing a null geodesic) - an important test of general relativity versus Newton. Divergent trajectories arising from gravitomagnetism (frame dragging, Lense-Thirring effect), gravitoelectric effects, geodetic effect, Shapiro delay, etc., do not contradict the equivalence principle.
Active, passive, and inertial masses
By definition of active and passive gravitational mass, the force on 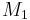 due to the gravitational field of
due to the gravitational field of 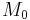 is:
is:
Likewise the force on a second object of arbitrary mass2 due to the gravitational field of mass0 is:
By definition of inertial mass:
If 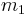 and
and 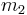 are the same distance
are the same distance  from
from 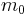 then, by the weak equivalence principle, they fall at the same rate (i.e. their accelerations are the same)
then, by the weak equivalence principle, they fall at the same rate (i.e. their accelerations are the same)
Hence:
Therefore:
In other words, passive gravitational mass must be proportional to inertial mass for all objects.
Furthermore by Newton's third law of motion:
must be equal and opposite to
It follows that:
In other words, passive gravitational mass must be proportional to active gravitational mass for all objects.
The dimensionless Eötvös-parameter  is the difference of the ratios of gravitational and inertial masses divided by their average for the two sets of test masses "A" and "B."
is the difference of the ratios of gravitational and inertial masses divided by their average for the two sets of test masses "A" and "B."
Tests of the weak equivalence principle
Tests of the weak equivalence principle are those that verify the equivalence of gravitational mass and inertial mass. An obvious test is dropping two contrasted objects in hard vacuum, e.g., inside Fallturm Bremen.
| Researcher | Year | Method | Result |
| John Philoponus | 6th century | Described correctly the effect of dropping balls of different masses | no detectable difference |
| Simon Stevin | ~1586 | Dropped lead balls of different masses off the Delft churchtower | no detectable difference |
| Galileo Galilei | ~1610 | Rolling balls down inclined planes | no detectable difference |
| Isaac Newton | ~1680 | measure the period of pendulums of different mass but identical length | no measurable difference |
| Friedrich Wilhelm Bessel | 1832 | measure the period of pendulums of different mass but identical length | no measurable difference |
| Loránd Eötvös | 1908 | measure the torsion on a wire, suspending a balance beam, between two nearly identical masses under the acceleration of gravity and the rotation of the Earth | difference is less than 1 part in 109 |
| Roll, Krotkov and Dicke | 1964 | Torsion balance experiment, dropping aluminum and gold test masses | 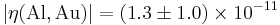 [3] [3] |
| David Scott | 1971 | Dropped a falcon feather and a hammer at the same time on the Moon | no detectable difference (not a rigorous experiment, but very dramatic being the first lunar one[4]) |
| Braginsky and Panov | 1971 | Torsion balance, aluminum and platinum test masses, measuring acceleration towards the sun | difference is less than 1 part in 1012 |
| Eöt-Wash group | 1987– | Torsion balance, measuring acceleration of different masses towards the earth, sun and galactic center, using several different kinds of masses | 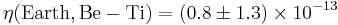 [5][6] [5][6] |
See:[7]
| Year | Investigator | Sensitivity | Method |
| 500? | Philoponus [8] | "small" | Drop Tower |
| 1585 | Stevin [9] | 5x10-2 | Drop Tower |
| 1590? | Galileo [10] | 2x10-2 | Pendulum, Drop Tower |
| 1686 | Newton [11] | 10-3 | Pendulum |
| 1832 | Bessel [12] | 2x10-5 | Pendulum |
| 1910 | Southerns [13] | 5x10-6 | Pendulum |
| 1918 | Zeeman [14] | 3x10-8 | Torsion Balance |
| 1922 | Eötvös [15] | 5x10-9 | Torsion Balance |
| 1923 | Potter [16] | 3x10-6 | Pendulum |
| 1935 | Renner [17] | 2x10-9 | Torsion Balance |
| 1964 | Dicke,Roll,Krotkov [18] | 3x10-11 | Torsion Balance |
| 1972 | Braginsky,Panov [19] | 10-12 | Torsion Balance |
| 1976 | Shapiro, et al.[20] | 10-12 | Lunar Laser Ranging |
| 1981 | Keiser,Faller [21] | 4x10-11 | Fluid Support |
| 1987 | Niebauer, et al.[22] | 10-10 | Drop Tower |
| 1989 | Heckel, et al.[23] | 10-11 | Torsion Balance |
| 1990 | Adelberger, et al.[24] | 10-12 | Torsion Balance |
| 1999 | Baeßler, et al.[25] | 5x10-13 | Torsion Balance |
| cancelled? | MiniSTEP | 10-17 | Earth Orbit |
| 2015? | MICROSCOPE | 10-16 | Earth Orbit |
Experiments are still being performed at the University of Washington which have placed limits on the differential acceleration of objects towards the Earth, the sun and towards dark matter in the galactic center. Future satellite experiments[26] – STEP (Satellite Test of the Equivalence Principle), Galileo Galilei, and MICROSCOPE (MICROSatellite pour l'Observation de Principe d'Equivalence) – will test the weak equivalence principle in space, to much higher accuracy.
The need to continue testing Einstein's theory of gravity may seem superfluous, as the theory is elegant and is compatible with almost all observations to date (except for instance the Pioneer anomaly). However, no quantum theory of gravity is known, and most suggestions violate one of the equivalence principles at some level. String theory, supergravity and even quintessence, for example, seem to violate the weak equivalence principle because they contain many light scalar fields with long Compton wavelengths. These fields should generate fifth forces and variation of the fundamental constants. There are a number of mechanisms that have been suggested by physicists to reduce these violations of the equivalence principle to below observable levels.
Proposed geometric test of the weak equivalence principle
Teleparallel gravity theory allows equivalence principle violation in a geometric parity Eötvös experiment. Spacetime geometry (chiral spacetime torsion as a vacuum left foot) is interrogated with test mass geometry (metaphoric opposite shoes) emergent at atomic bond distances. A difference in fit appears as non-identical minimum action vacuum free fall trajectories (divergent in path and/or rate) - an equivalence principle violation.
There is no boson photon vacuum refraction, dispersion (optics), dichroism, or gyrotropy even for billion lightyear pathlengths.[27] Nobody knows if the vacuum is measurably anisotropic toward resolved opposite chirality fermion atomic mass distributions.
Laboratory equivalence principle tests contrast net residual divergences of chemical composition, spin (angular momentum: mechanical;[28][29][30] quantum particle spin and quantum orbital [31]), nuclear binding energy, etc. Eötvös experiments detect no composition or field equivalence principle violations to 5x10-14 difference/average.[32] Lunar laser ranging observes zero Nordtvedt effect. Astronomic pulsar binary star systems allow strong field extreme physical, compositional, relativistic, and quantum mechanical contrasts heroically beyond local measurements.
1.74 solar-mass 465.1 Hz pulsar PSR J1903+0327 plus a 1.05 solar-mass star are a 95.17-day orbit binary system.[33] 15.3% (AP4 model radius);[34][35][36] vs. 0.0001% gravitational binding energy, 1.8x1011 vs. 30 surface gees, 2x108 gauss vs. 5 gauss magnetic field; compressed superfluid neutrons and superconductive protons [37] vs. proton-electron plasma, extreme isospin and lepton number divergence; and pulsar 11% (AP4) of lightspeed equatorial spin velocity are differentially EP-inert for orbit, apsidal precession, and gravitational radiation orbital decay. 1.97 solar-mass 317.5 Hz PSR J1614-2230 and a 0.5 solar-mass He-C-O white dwarf contrast the pulsar with Fermi-degenerate matter, 20% versus 0.01% gravitational binding energy,[38] to no detected equivalence principle anomaly.
No measurable observable violates the equivalence principle in laboratory, local, and astronomic contrasts. Atomic mass distribution geometric parity (chirality in all directions) can be observed and calculated [39] but it cannot be measured. (Specific rotation of plane-polarized light is an electronic effect that does not measure atomic mass distribution chirality.[40] It is unknown if (metaphoric) opposite shoes violate the equivalence principle.
Crystallography affords 230 unique ways to arrange points in three dimensions, the space groups. 11 pairs of enantiomorphic space groups are mathematical geometric parity (physics) pairs independent of composition. Delete individual space groups containing racemic or opposing sense screw axes. There remain enantiomorphic space groups P3121 | P3221 (e.g., alpha-quartz) and P31 | P32 (e.g., gamma-glycine). A geometric parity Eötvös experiment opposes chemically and macroscopically identical, left-handed versus right-handed atomic mass distributions as single crystal test masses - opposite shoes emergent at atomic bond lengths. The Eötvös apparatus and experimental protocol are otherwise unchanged.[41]
Calorimetry detects geometric parity violation 40 times smaller than a torsion balance composition violation.[42] Enthalpies of fusion of enantiomorphic space groups P3121 and P3221 benzil single crystals[43] are simultaneously determined in paired differential scanning calorimeters to OEM 0.1% precision. That corresponds to 1.3x10−15 difference/average torsion balance sensitivity. Secondary standard 95°C calibration of calorimeters with powdered racemic benzil tolerates a 10−12 relative signal for resolved single crystals. Paired measurements on new crystals made each 30 minutes for 24 hours detect both vacuum insertion energy differences and inertial acceleration (Earth's spin) versus gravitational acceleration (Earth's orbit) varying chirality alignments[44] - Lorentz invariance and equivalence principle violations.
The Einstein equivalence principle
The Einstein equivalence principle states that the weak equivalence principle holds, and that:[45]
- The outcome of any local non-gravitational experiment in a freely falling laboratory is independent of the velocity of the laboratory and its location in spacetime.
Here "local" has a very special meaning: not only must the experiment not look outside the laboratory, but it must also be small compared to variations in the gravitational field, tidal forces, so that the entire laboratory is freely falling. It also implies the absence of interactions with "external" fields other than the gravitational field.
The principle of relativity implies that the outcome of local experiments must be independent of the velocity of the apparatus, so the most important consequence of this principle is the Copernican idea that dimensionless physical values such as the fine-structure constant and electron-to-proton mass ratio must not depend on where in space or time we measure them. Many physicists believe that any Lorentz invariant theory that satisfies the weak equivalence principle also satisfies the Einstein equivalence principle.
Schiff's conjecture suggests that the weak equivalence principle actually implies the Einstein equivalence principle, but it has not been proven. Nonetheless, the two principles are tested with very different kinds of experiments. The Einstein equivalence principle has been criticized as imprecise, because there is no universally accepted way to distinguish gravitational from non-gravitational experiments (see for instance Hadley[46] and Durand[47]).
Tests of the Einstein equivalence principle
In addition to the tests of the weak equivalence principle, the Einstein equivalence principle can be tested by searching for variation of dimensionless constants and mass ratios. The present best limits on the variation of the fundamental constants have mainly been set by studying the naturally occurring Oklo natural nuclear fission reactor, where nuclear reactions similar to ones we observe today have been shown to have occurred underground approximately two billion years ago. These reactions are extremely sensitive to the values of the fundamental constants.
| Constant | Year | Method | Limit on fractional change |
| fine structure constant | 1976 | Oklo | 10−7 |
| weak interaction constant | 1976 | Oklo | 10−2 |
| electron-proton mass ratio | 2002 | quasars | 10−4 |
| proton gyromagnetic factor | 1976 | astrophysical | 10−1 |
There have been a number of controversial attempts to constrain the variation of the strong interaction constant. There have been several suggestions that "constants" do vary on cosmological scales. The best known is the reported detection of variation (at the 10−5 level) of the fine-structure constant from measurements of distant quasars, see Webb et al.[48] Other researchers dispute these findings. Other tests of the Einstein equivalence principle are gravitational redshift experiments, such as the Pound-Rebka experiment which test the position independence of experiments.
The strong equivalence principle
The strong equivalence principle suggests the laws of gravitation are independent of velocity and location. In particular,
- The gravitational motion of a small test body depends only on its initial position in spacetime and velocity, and not on its constitution.
and
- The outcome of any local experiment (gravitational or not) in a freely falling laboratory is independent of the velocity of the laboratory and its location in spacetime.
The first part is a version of the weak equivalence principle that applies to objects that exert a gravitational force on themselves, such as stars, planets, black holes or Cavendish experiments. The second part is the Einstein equivalence principle (with the same definition of "local"), restated to allow gravitational experiments and self-gravitating bodies. The freely-falling object or laboratory, however, must still be small, so that tidal forces may be neglected (hence "local experiment").
This is the only form of the equivalence principle that applies to self-gravitating objects (such as stars), which have substantial internal gravitational interactions. It requires that the gravitational constant be the same everywhere in the universe and is incompatible with a fifth force. It is much more restrictive than the Einstein equivalence principle.
The strong equivalence principle suggests that gravity is entirely geometrical by nature (that is, the metric alone determines the effect of gravity) and does not have any extra fields associated with it. If an observer measures a patch of space to be flat, then the strong equivalence principle suggests that it is absolutely equivalent to any other patch of flat space elsewhere in the universe. Einstein's theory of general relativity (including the cosmological constant) is thought to be the only theory of gravity that satisfies the strong equivalence principle. A number of alternative theories, such as Brans-Dicke theory, satisfy only the Einstein equivalence principle.
Tests of the strong equivalence principle
The strong equivalence principle can be tested by searching for a variation of Newton's gravitational constant G over the life of the universe, or equivalently, variation in the masses of the fundamental particles. A number of independent constraints, from orbits in the solar system and studies of big bang nucleosynthesis have shown that G cannot have varied by more than 10%.
Thus, the strong equivalence principle can be tested by searching for fifth forces (deviations from the gravitational force-law predicted by general relativity). These experiments typically look for failures of the inverse-square law (specifically Yukawa forces or failures of Birkhoff's theorem) behavior of gravity in the laboratory. The most accurate tests over short distances have been performed by the Eöt-Wash group. A future satellite experiment, SEE (Satellite Energy Exchange), will search for fifth forces in space and should be able to further constrain violations of the strong equivalence principle. Other limits, looking for much longer-range forces, have been placed by searching for the Nordtvedt effect, a "polarization" of solar system orbits that would be caused by gravitational self-energy accelerating at a different rate from normal matter. This effect has been sensitively tested by the Lunar Laser Ranging Experiment. Other tests include studying the deflection of radiation from distant radio sources by the sun, which can be accurately measured by very long baseline interferometry. Another sensitive test comes from measurements of the frequency shift of signals to and from the Cassini spacecraft. Together, these measurements have put tight limits on Brans-Dicke theory and other alternative theories of gravity.
Challenges to the equivalence principle
The best known challenge to the equivalence principle is the Brans-Dicke theory; however, the theory represents the viewpoint of a tiny minority in the physics community. Self-creation cosmology is a modification of the Brans-Dicke theory. The Fredkin Finite Nature Hypothesis is an even more radical challenge to the equivalence principle and has even fewer supporters.
In August 2010, researchers from the School of Physics, University of New South Wales, Australia; the Centre for Astrophysics and Supercomputing, Swinburne University of Technology, Australia; and the Institute of Astronomy, Cambridge, United Kingdom; published the paper "Evidence for spatial variation of the fine structure constant", whose tentative conclusion is that, "qualitatively, [the] results suggest a violation of the Einstein Equivalence Principle, and could infer a very large or infinite universe, within which our `local' Hubble volume represents a tiny fraction."[49]
Explanations of the equivalence principle
Dutch physicist and string theorist Erik Verlinde has generated a self-contained, logical derivation of the equivalence principle based on the starting assumption of a holographic universe. Given this situation, gravity would not be a true fundamental force as is currently thought but instead an "emergent property" related to entropy. Verlinde's approach to explaining gravity apparently leads naturally to the correct observed strength of dark energy; previous failures to explain its incredibly small magnitude have been called "the greatest embarassment in the history of theoretical physics". However, it should be noted that these ideas are far from settled and still very controversial.
The equivalence principle guarantees that a gravity field (a central force) cannot be distinguished from forces due to uniform acceleration. If two balls are dropped toward a gravitating mass the balls will converge as they fall towards the mass centroid. Under uniform acceleration, such as the ubiquitous accelerating elevator, the dropped balls will not converge, but fall in parallel paths.
Experiments
- University of Washington[50]
- Lunar Laser Ranging[51]
- Galileo-Galilei satellite experiment[52]
- Satellite Test of the Equivalence Principle (STEP)[53]
- MICROSCOPE[54]
- Satellite Energy Exchange (SEE)[55]
- "...Physicists in Germany have used an atomic interferometer to perform the most accurate ever test of the equivalence principle at the level of atoms..."[56]
See also
Notes
- ^ A. Einstein. “How I Constructed the Theory of Relativity,” Translated by Masahiro Morikawa from the text recorded in Japanese by Jun Ishiwara, Association of Asia Pacific Physical Societies (AAPPS) Bulletin, Vol. 15, No. 2, pp. 17-19 (April 2005). Einstein recalls events of 1907 in talk in Japan on 14 December 1922.
- ^ Paul S Wesson (2006). Five-dimensional Physics. World Scientific. p. 82. ISBN 9812566619. http://books.google.com/?id=dSv8ksxHR0oC&printsec=frontcover&dq=intitle:Five+intitle:Dimensional+intitle:Physics.
- ^ P. G. Roll, R. Krotkov, R. H. Dicke, The equivalence of inertial and passive gravitational mass, Annals of Physics, Volume 26, Issue 3, 20 February 1964, Pages 442-517
- ^ http://www.youtube.com/watch?v=MJyUDpm9Kvk
- ^ Schlamminger, S.; Choi, K.-Y.; Wagner, T.; Gundlach, J.; Adelberger, E. (2008). "Test of the Equivalence Principle Using a Rotating Torsion Balance". Physical Review Letters 100 (4). Bibcode 2008PhRvL.100d1101S. doi:10.1103/PhysRevLett.100.041101.
- ^ Schlamminger; Choi; Wagner; Gundlach; Adelberger (2007). "Test of the Equivalence Principle Using a Rotating Torsion Balance". Phys.Rev.Lett.100:041101,2008 100 (4). arXiv:0712.0607. Bibcode 2008PhRvL.100d1101S. doi:10.1103/PhysRevLett.100.041101.
- ^ Ciufolini & Wheeler, "Gravitation and Inertia" (Princeton University Press: Princeton, 1995) pp. 117-119
- ^ Philoponus, J. "Corollaries on Place and Void" David Furley trans. (Ithaca, NY: Cornell University Press, 1987)
- ^ Stevin, Simon. De Beghinselen der Weeghconst "Principles of the Art of Weighing" (Leyden, 1586); Dijksterhuis, EJ. "The Principal Works of Simon Stevin" (Amsterdam 1955)
- ^ Galilei, Galileo. "Discorsi e Dimostrazioni Matematiche Intorno a Due Nuove Scienze" (Appresso gli Elsevirii, Leida: 1638); "Discourses and Mathematical Demonstrations Concerning Two New Sciences," (Elsevier Press, Leiden: Netherlands, 1638)
- ^ Newton, Isaac. "Philosophiae Naturalis Principia Mathematica" (Mathematical Principles of Natural Philosophy and his System of the World), trans. by A. Motte and revised by F. Cajori (University of California Press: Berkeley, 1934); Newton, Isaac "The Principia: Mathematical Principles of Natural Philosophy" Trans. I. Bernard Cohen and Anne Whitman, with the assistance of Julia Budenz (University of California Press: Berkeley, 1999)
- ^ Ann. Physik und Chemie (Poggendorff) 25 401 (1832)
- ^ Proc. Roy. Soc. Lond. 84 325 (1910)
- ^ Proc. K. Akad. Amsterdam 20(4) 542 (1918)
- ^ Math. Naturw. Ber. aus. Ungarn 8 65 (1889); Ann. Physik (Leipzig) 68 11 (1922); Phys. Rev. D 61(2) 022001 (1999)
- ^ Proc. Roy. Soc. Lond. 104 588 (1923)
- ^ és Természettudományi Értesitö 53 569 (1935)
- ^ Ann. Phys. (NY) 26 442 (1964)
- ^ Zh. Eksp. Teor. Fiz. 61 873 (1971)
- ^ Phys. Rev. Lett. 36 555 (1976)
- ^ Bull. Am. Phys. Soc. 24 579 (1979)
- ^ Phys. Rev. Lett. 59 609 (1987)
- ^ Phys. Rev. Lett. 62 609 (1989)
- ^ Phys. Rev. D 42 3267 (1990)
- ^ Class. Quantum. Grav. 18(13) 2393 (2001); Phys.Rev. Lett 83(18) 3585 (1999)
- ^ Dittus, H; C. Lāmmerzahl. "Experimental Tests of the Equivalence Principle and Newton’s Law in Space" (PDF). GRAVITATION AND COSMOLOGY: 2nd Mexican Meeting on Mathematical and Experimental Physics. AIP Conference Proceedings 758: 95. Bibcode 2005AIPC..758...95D. doi:10.1063/1.1900510. http://www.zarm.uni-bremen.de/2forschung/gravi/publications/papers/2005DittusLaemmerzahl.pdf.
- ^ and 0905.1929, 0706.2031, 1106.1068
- ^ Z.B. Zhou, J. Luo, Q. Yan, Z.G. Wu, Y.Z. Zhang, and Y.X. Nie, "New upper Limit of terrestrial equivalence principle test for rotating extended bodies" Phys. Rev. D66 022002 (2002) [arxiv:hep-ph/0303143]
- ^ J. Luo, Y.X. Nie, Y.Z. Zhang, and Z.B. Zhou, "Null result for the violation of equivalence Principle with free-fall rotating gyroscopes" Phys.Rev. D65 042005 (2002) [arxiv:gr-qc/0111069]
- ^ Y.Z. Zhang, J. Luo, and Y.X. Nie, "Gravitational effects of rotating bodies" Mod. Phys. Lett. A 16 789 (2001)[arxiv:gr-qc/0006075]
- ^ B. R. Heckel, C. E. Cramer, T. S. Cook, E. G. Adelberger, S. Schlamminger, and U. Schmidt, "New CP-Violation and Preferred-Frame Tests with Polarized Electrons" Phys. Rev. Lett. 97 021603 (2006) [ariv:0808.2673]
- ^ E. G. Adelberger, J. H. Gundlach, B. R. Heckel, S. Hoedl and S. Schlamminger, "Torsion balance experiments: a low-energy frontier of particle physics" Prog. Part. Nucl. Phys. 62 102 (2009)
- ^ D. J. Champion, S. M. Ransom, P. Lazarus, F. Camilo, C. Bassa, V. M. Kaspi, et al., "An Eccentric Binary Millisecond Pulsar in the Galactic Plane" Science 320(5881) 1309 (2004) [arxiv:0805.2396]
- ^ J. M. Lattimer and M. Prakash "Neutron star structure and the equation of state" Astrophysical J. 550(1) 426 (2001) [arxiv:astro-ph/0002232]
- ^ A. Akmal, V. R. Pandharipande, D. G. Ravenhall, "The equation of state for nucleon matter and neutron star structure" Phys. Rev. C 58(4) 1804 (1999) [ariv:nucl-th/9804027]
- ^ C. J. Pethick, A. Akmal, V. R. Pandharipande, D. G. Ravenhall, "Neutron Star Structure" [arxiv:astro-ph/9905177]
- ^ D. Page, M. Prakash, J. M. Lattimer, and A. W. Steiner, "Rapid Cooling of the Neutron Star in Cassiopeia A Triggered by Neutron Superfluidity in Dense Matter" Phys. Rev. Lett. 106 081101 (2011) [arxiv:1011.6142]
- ^ P. Demorest, T. Pennucci, S. Ransom, M. Roberts, and J. Hessels, "Shapiro delay measurement of a two solar mass neutron star" Nature 467 1081 (2010) [arxiv:1010.5788]
- ^ M. Petitjean, "On the root mean square quantitative chirality and quantitative symmetry measures" J. Math. Phys. 40 4587 (1999); http://petitjeanmichel.free.fr/itoweb.petitjean.html; http://petitjeanmichel.free.fr/itoweb.petitjean.freeware.html#QCM
- ^ then N. A. Saccomano, US Pat. No. 5395935, March 1995; T. D. Crawford, M. C. Tam, and M. L. Abrams, J. Phys. Chem. A 111(48) 12057 (2007); K. Wiberg, Y-G Wang, S. Wilson, P. Vaccaro, and J. R. Cheeseman, J. Phys. Chem. A 110(51) 13995 (2006); R. N. Patel, "Enzymatic preparation of chiral pharmaceutical intermediates by lipases" J. Liposome Res. 11(4) 355 (2001)
- ^ http://www.mazepath.com/uncleal/erotor1.jpg
- ^ http://www.mazepath.com/uncleal/shoes2.png
- ^ http://www.mazepath.com/uncleal/benzil.png
- ^ http://www.mazepath.com/uncleal/orbit.png
- ^ Haugen, Mark P.; C. Lämmerzahl (2001). Principles of Equivalence: Their Role in Gravitation Physics and Experiments that Test Them. Springer. arXiv:gr-qc/0103067. ISBN 978-3-540-41236-6.
- ^ Hadley (1997). "The Logic of Quantum Mechanics Derived from Classical General Relativity". Found.Phys.Lett. 10 (1997) 43-60 10: 43–60. arXiv:quant-ph/9706018. Bibcode 1997FoPhL..10...43H. doi:10.1007/BF02764119.
- ^ http://stacks.iop.org/ob/4/S351
- ^ Webb; Murphy; Flambaum; Dzuba; Barrow; Churchill; Prochaska; Wolfe (2000). "Further Evidence for Cosmological Evolution of the Fine Structure Constant". Phys.Rev.Lett.87:091301,2001 87 (9). arXiv:astro-ph/0012539. Bibcode 2001PhRvL..87i1301W. doi:10.1103/PhysRevLett.87.091301. PMID 11531558.
- ^ Webb; King; Murphy; Flambaum; Carswell; Bainbridge (2010). "Evidence for spatial variation of the fine structure constant". arXiv:1008.3907 [astro-ph.CO].
- ^ Eöt-Wash group
- ^ http://funphysics.jpl.nasa.gov/technical/grp/lunar-laser.html
- ^ http://eotvos.dm.unipi.it/nobili/
- ^ http://einstein.stanford.edu/STEP/
- ^ http://smsc.cnes.fr/MICROSCOPE/index.htm
- ^ http://www.phys.utk.edu/see/
- ^ 16 November 2004, physicsweb: Equivalence principle passes atomic test
References
- R. H. Dicke, "New Research on Old Gravitation," Science 129, 3349 (1959). This paper is the first to make the distinction between the strong and weak equivalence principles.
- R. H. Dicke, "Mach's Principle and Equivalence," in Evidence for gravitational theories: proceedings of course 20 of the International School of Physics "Enrico Fermi", ed C. Møller (Academic Press, New York, 1962). This article outlines the approach to precisely testing general relativity advocated by Dicke and pursued from 1959 onwards.
- Albert Einstein, "Über das Relativitätsprinzip und die aus demselben gezogene Folgerungen," Jahrbuch der Radioaktivitaet und Elektronik 4 (1907); translated "On the relativity principle and the conclusions drawn from it," in The collected papers of Albert Einstein. Vol. 2 : The Swiss years: writings, 1900–1909 (Princeton University Press, Princeton, NJ, 1989), Anna Beck translator. This is Einstein's first statement of the equivalence principle.
- Albert Einstein, "Über den Einfluß der Schwerkraft auf die Ausbreitung des Lichtes," Annalen der Physik 35 (1911); translated "On the Influence of Gravitation on the Propagation of Light" in The collected papers of Albert Einstein. Vol. 3 : The Swiss years: writings, 1909–1911 (Princeton University Press, Princeton, NJ, 1994), Anna Beck translator, and in The Principle of Relativity, (Dover, 1924), pp 99–108, W. Perrett and G. B. Jeffery translators, ISBN 0-486-60081-5. The two Einstein papers are discussed online at The Genesis of General Relativity.
- C. Brans, "The roots of scalar-tensor theory: an approximate history", arXiv:gr-qc/0506063. Discusses the history of attempts to construct gravity theories with a scalar field and the relation to the equivalence principle and Mach's principle.
- C. W. Misner, K. S. Thorne and J. A. Wheeler, Gravitation, W. H. Freeman and Company, New York (1973), Chapter 16 discusses the equivalence principle.
- Hans Ohanian and Remo Ruffini Gravitation and Spacetime 2nd edition, Norton, New York (1994). ISBN 0-393-96501-5 Chapter 1 discusses the equivalence principle, but incorrectly, according to modern usage, states that the strong equivalence principle is wrong.
- J. P. Uzan, "The fundamental constants and their variation: Observational status and theoretical motivations," Rev. Mod. Phys. 75, 403 (2003). arXiv:hep-ph/0205340 This technical article reviews the best constraints on the variation of the fundamental constants.
- C. M. Will, Theory and experiment in gravitational physics, Cambridge University Press, Cambridge (1993). This is the standard technical reference for tests of general relativity.
- C. M. Will, Was Einstein Right?: Putting General Relativity to the Test, Basic Books (1993). This is a popular account of tests of general relativity.
- C. M. Will, The Confrontation between General Relativity and Experiment, Living Reviews in Relativity (2006). An online, technical review, covering much of the material in Theory and experiment in gravitational physics. The Einstein and strong variants of the equivalence principles are discussed in sections 2.1 and 3.1, respectively.
- Michael Friedman, Foundations of Space-Time Theories, Princeton University Press, Princeton (1983). Chapter V discusses the equivalence principle.
External links
- Equivalence Principle at NASA, including tests
- Introducing The Einstein Principle of Equivalence from Syracuse University
- The Equivalence Principle at MathPages
- The Einstein Equivalence Principle at Living Reviews on General Relativity
- http://cafe.daum.net/grelativitycosmology